Demystifying Karnaugh Maps: A Comprehensive Guide To Five-Variable Simplification

Demystifying Karnaugh Maps: A Comprehensive Guide to Five-Variable Simplification

Related Articles: Demystifying Karnaugh Maps: A Comprehensive Guide to Five-Variable Simplification

Introduction

In this auspicious occasion, we are delighted to delve into the intriguing topic related to Demystifying Karnaugh Maps: A Comprehensive Guide to Five-Variable Simplification. Let’s weave interesting information and offer fresh perspectives to the readers.

Demystifying Karnaugh Maps: A Comprehensive Guide to Five-Variable Simplification

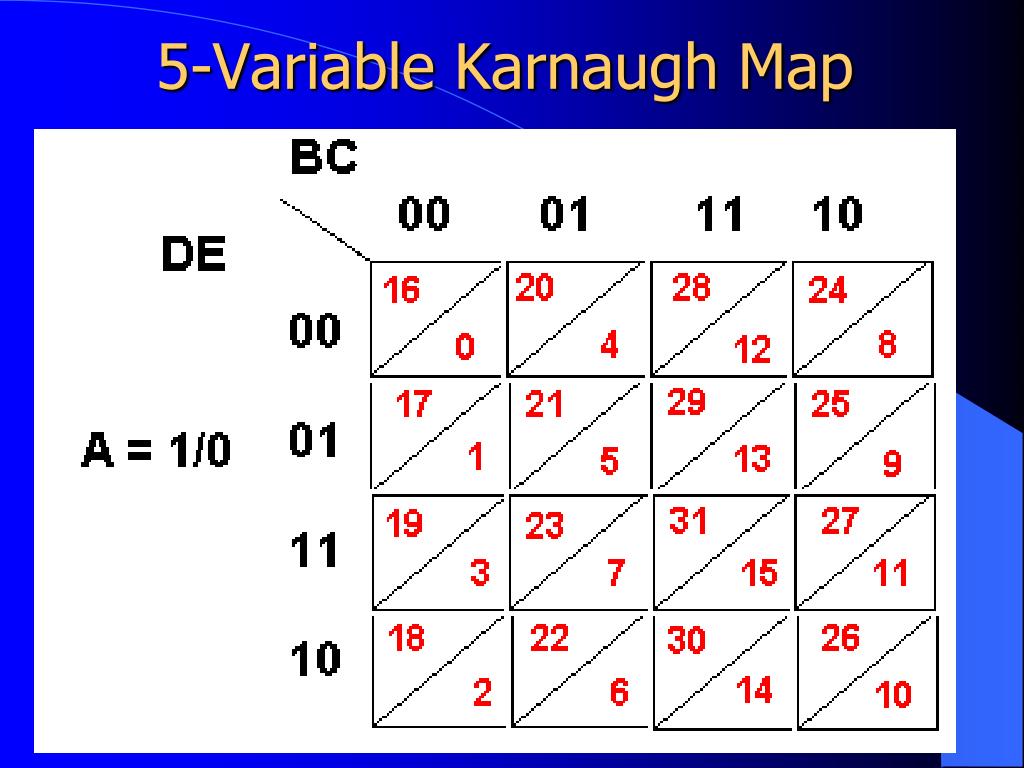
The Karnaugh map, or K-map, is a powerful tool used in digital logic design to simplify Boolean expressions and minimize logic circuits. While commonly employed for expressions with up to four variables, K-maps can be extended to handle five variables, albeit with a slight increase in complexity. This article delves into the intricacies of five-variable K-maps, providing a comprehensive understanding of their structure, implementation, and benefits.

Understanding the Structure of a Five-Variable K-Map

A five-variable K-map is essentially a two-dimensional representation of a truth table, organized in a specific way to facilitate the identification of adjacent minterms (or maxterms) that can be combined for simplification. It consists of a 3D structure, where two dimensions represent four variables (A, B, C, D) and the third dimension represents the fifth variable (E).

The four-variable map is presented as a standard 2×4 or 4×4 grid, with each cell representing a unique combination of the variables A, B, C, and D. The fifth variable (E) is then incorporated by creating two identical copies of this four-variable map, one for E=0 and the other for E=1. These two maps are placed side-by-side, visually representing the third dimension.

Visualizing the Five-Variable K-Map

Imagine two four-variable K-maps stacked on top of each other, with the top map representing E=0 and the bottom map representing E=1. Each cell in the top map corresponds to a cell in the bottom map, forming a vertical "column" of cells. This column represents a unique combination of the five variables.

Grouping and Simplification

The primary goal of using a K-map is to simplify Boolean expressions by grouping adjacent minterms (or maxterms). In a five-variable K-map, adjacency can occur in three different ways:

1. Horizontal and Vertical Adjacency: Minterms within the same four-variable map are adjacent if they differ by only one variable. This is similar to the traditional K-map grouping rules.

2. Column Adjacency: Minterms in the corresponding cells of the two four-variable maps (E=0 and E=1) are considered adjacent, as they differ only in the value of the fifth variable (E).

3. Wrap-Around Adjacency: Similar to four-variable maps, adjacency can wrap around the edges of the four-variable maps. For example, the leftmost column in the top map is adjacent to the rightmost column in the same map.

Example: Simplifying a Five-Variable Expression

Let’s consider the following Boolean expression:

F(A, B, C, D, E) = Σ(0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 16, 17, 18, 19, 24, 25, 26, 27)

To simplify this expression using a five-variable K-map, we follow these steps:

1. Create the K-map: Construct two identical four-variable K-maps, one for E=0 and the other for E=1.

2. Populate the K-map: Enter "1" in the cells corresponding to the minterms listed in the expression. For example, minterm 0 (A=0, B=0, C=0, D=0, E=0) would be placed in the top left corner of the E=0 map.

3. Identify Groups: Look for groups of adjacent "1"s. The groups can be of size 1, 2, 4, 8, or 16. Remember to consider both horizontal/vertical and column adjacency, as well as wrap-around.

4. Write the Simplified Expression: For each group, write a product term corresponding to the variables that remain constant within the group. For example, a group of four "1"s in the top left corner of the E=0 map would represent the product term ‘A’B’C’D’.

5. Combine the Terms: Sum all the product terms obtained from the groups to form the simplified expression.

Benefits of Using Five-Variable K-Maps

• Simplified Logic Circuits: K-maps effectively minimize the number of logic gates required to implement a Boolean expression, resulting in a more efficient and cost-effective circuit.

• Improved Readability and Understanding: K-maps provide a visual representation of the Boolean expression, making it easier to understand the relationships between variables and identify potential simplifications.

• Systematic Approach: The K-map method offers a structured and systematic approach to Boolean expression simplification, ensuring a consistent and reliable outcome.

FAQs about Five-Variable K-Maps

Q1: What is the maximum number of variables that can be represented using a K-map?

A: Theoretically, K-maps can be extended to represent any number of variables. However, the size and complexity of the map increase exponentially with the number of variables. In practice, K-maps are typically used for expressions with up to six variables, beyond which alternative simplification techniques become more practical.

Q2: Can K-maps be used for simplifying expressions with don’t care conditions?

A: Yes, K-maps can effectively handle don’t care conditions. Don’t care conditions are represented by "X" in the K-map. They can be included in groups to further simplify the expression, as they can be treated as either "0" or "1" depending on what leads to a simpler expression.

Q3: What are some limitations of using K-maps?

A: While powerful, K-maps have some limitations:

• Complexity: The size and complexity of the map increase rapidly with the number of variables, making it difficult to handle expressions with more than six variables.

• Visual Representation: K-maps rely on visual interpretation, which can be subjective and prone to errors, especially for complex expressions.

• Limited Flexibility: K-maps are best suited for simplifying expressions with a specific structure, and may not be as effective for more complex or non-standard expressions.

Tips for Using Five-Variable K-Maps Effectively

• Organize the K-map: Ensure the variables are arranged in a consistent order within the map to avoid confusion and errors.

• Use a Systematic Approach: Follow a structured process for identifying groups, writing product terms, and combining them to form the simplified expression.

• Check for Adjacency: Carefully examine the map for all possible adjacencies, including column adjacency and wrap-around, to maximize simplification.

• Don’t Overlook Don’t Cares: Utilize don’t care conditions effectively to further simplify the expression.

• Verify the Result: After simplifying the expression, verify the result by comparing it to the original expression or by using a truth table.

Conclusion

Five-variable Karnaugh maps provide a valuable tool for simplifying Boolean expressions and designing efficient logic circuits. While requiring a slightly more complex approach than their four-variable counterparts, they offer a clear and systematic method for identifying adjacent minterms (or maxterms) and deriving simplified expressions. By understanding the structure, implementation, and benefits of five-variable K-maps, designers can effectively leverage this tool to optimize digital logic designs, leading to improved performance, reduced cost, and enhanced circuit complexity.

Closure

Thus, we hope this article has provided valuable insights into Demystifying Karnaugh Maps: A Comprehensive Guide to Five-Variable Simplification. We appreciate your attention to our article. See you in our next article!